RBSE Solutions for Class 12 Maths Chapter 16 Probability and Probability Distribution Miscellaneous Exercise

RBSE Solutions for Class 12 Maths Chapter 16 Probability and Probability Distribution Miscellaneous Exercise

Rajasthan Board RBSE Class 12 Maths Chapter 16 Probability and Probability Distribution Miscellaneous Exercise

Question 1.
Two events A and B are said to be mutually independent, if:

Solution:
Given A and B two independent events

Hence option (iii) is correct

Question 2.
Two dice are thrown. The probability of getting a pair of even prime number is :
(i) 1/3
(ii) 0
(iii) 1/36
(iv) 1/12
Solution:
Probability of getting 2 even prime number on one dice = 1/6
Probability of getting 2 an even number on other dice = 1/6
∴ Probability that 2 even prime number is found when a pair of dice is tossed
= 1/6 × 1/6 = 1/36
Hence option (iii) is correct.

Question 3.
If A and B be two events, such that A ⊂ B and P(B) = 0, then which of the following statement is true ?

Solution :

Question 4.
Two cards are drawn randomly from a well- shuffled deck of 52 cards. If X denotes the number of aces, then find mean of X:

Solution:
Two cards are drawn randomly from 52 cards, firstly number of total ways that two cards are not an ace.

Number of ways drawing 2 cards out of 52 cards

∴ P(X = 0), Probability that no ace is not drawn = 1128/1326
Secondly number of ways that there is an ace and there is no ace out of ⁴C₁ × ⁴⁸C₁


Hence option (iv) is correct

Question 5.
A random variable X, takes the values 0,1,2, and 3. Mean of X is P(x = 3) = 2P(x = 1) and P(x = 2) = 0-3, then P(X=0) is :
(i) 0.2
(ii) 0.4
(iii) 0.3
(iv) 0.1
Solution:

Question 6.
Probability of girl to be a racer is 4/5 What is the probability that 4 girls out of 5 are racer ?

Solution:
Probability that girl to eb a racer = 4/5
∴ Probability that girls in not be racer
= 1 – 4/5 = 1/5
∴ Probability distribution of girls to be racer
= (4/5 + 1/5)⁵
Probability that 4 girls are to be racer
= ⁵C₄ (45)⁴ × (15)
Hence option (iii) is correct

Question 7.
In a box containing 100 are defective. The probability that out of a sample of 5 bulbs, none is defective is :

Solution:
Number of bulbs in box = 100
Number of defective bulbs = 10
∴ Probability of defective bulbs = 10/100 = 1/10
∴ So probability of non defective bulb = 1 – 1/10 = 9/10
∴ Probability that no bulb is defective out a sample of 5

Hence option (iv) is correct

Question 8.
A couple has two children. Find the probability that
(i) both children are male, if it is known that the elder child is a male.
(ii) both children are female, it it is known that the elder child is a female.
(iii) both children are male, if it is known that at least one of the children is male.
Solution:

Question 9.
Two integers are selected at random from the 1 to 11 integers. Find the probability that both integers obtained are odd, if it is known that the sum of two numbers is even.
Solution:
There are 3 even numbers and 6 odd numbers between the numbers 1 to 11
Let A = Event that two odd numbers are choosen
and B = Event that sum of two numbers is even.
n(A) = number of ways to choose 2 odd numbers out of 6 odd numbers = ⁶C₂
n(B) = Number of ways to choose two numbers whose sum is even = ⁵C₂ + ⁵C₂
∴ n(A ∩ B) = Number of ways to choose 2 odd numbers whose sum is even = ⁶C₂
Let sample space be S
∴ n(S) = number of ways to choose two
numbers out of 11 numbers = ¹¹C₂

Question 10.
An electronic assembly consists of two subsystem say, A and B. From previous testing procedures, the following probabilities are assumed to be known :
P (A fails) = 0.2
P (B fails alone) = 0.15
P (A and B fail) = 0.15
Evaluate the following probabilities
(i) P (A fails/B has failed)
(ii) P (A fails alone).
Solution:

Question 11.
Let A and B be two independent events. The probability of their simultanous occurrence is 1/8 and the probability that neither occurs 3/8 Find P(A) and P(B).
Solution:
A and B are independent events.

Question 12.
Anil speaks truth in 60% of cases and Anand in 90% of the cases. In what percentage of cases are the likely to contradict each other in stating the same fact ?
Solution:

Question 13.
Three persons A, B, C in order toss a coin. The one to throw a head wins. What are their respective chances of winning assuming that the game may continue indefinitely ?
Solution:
Chances to be head on tossing a coin = 1/2
∵ A starts to play, then A will play again on fourth, seventh ………. then can win.
∴ Possibilities that A wins

Question 14.
Probability of a man to live for next 25 years is 4/5 and so, his wife is 3/4, Find the probabilities that
(i) both live for 25 years
(ii) at least one of them live for 25 years
(iii) Only wife live for 25 years.
Solution :
Let A : Event that man live for next 25 years and
B : event that wife live for next 25 years.
Clearly both are independent events

Question 15.
In three groups of children there are 3 girls and 1 boy, 2 girls, 2 boys, 1 girl and 3 boys. A child is randomly selected from each group. Find the probability that selected children are 1 girl and 2 boys.
Solution:
G^I, G^II and G^III are three groups of students. Then 1 girl and 2 boys can be selected in following ways.

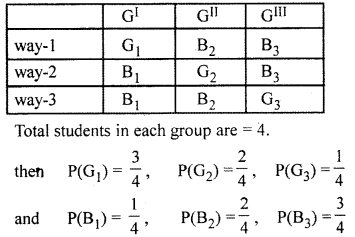
Here Girls and Boys are represented by G₁, G₂, G₃ and B₁, B₂, B₃ resp. Hence required probability is
= P (1 Girl and 2 boys are relected)

Question 16.
Bag I contains 3 black and 4 white balls and bag II contains 4 black and 3 white balls. Now an unbiased die throws, if number 1 or 3 shows on die then a ball is drawn from bag I otherwise from bag II. Find the probability that the ball so drawn is black.
Solution:
Number of total balls in I bag 3 + 4 = 7, where 3 are black and 4 are white.
Number of balls in II bag = 4 + 3 = 7, where 4 are black and 3 are white.
Total number of result on throwing a die S = {1,2, 3,4, 5, 6}
Let E₁ be the event to get 1 and 3

Question 17.
A person has undertaken a construction job. The probabilities are 0.65 that there will be strike, 0.80 that the construction job will be completed on time if there is no strike and 0.32 that the construction job will be completed on time if there is a strike. Determine the probability that the construction job will be completed on time.
Solution:
Probability that there will be strike
P(A) = 0.65
Probability that there will not be strike
P() = 1 – 0.65 = 0.35
Let E the event that work finished in time.
Then probability of completion of work in strike.
P (E/A) = 0.32
and probability of completion of work when there is no strike
P (E/A) = 0.80
∴ Probability that the construction job will be completed on time

Question 18.
Bag I contains 8 white and 7 black balls and bag II contains 5 white and 4 black balls. One ball is randomly transferred from bag I to bag II. Then a ball is drawn from bag II. Find the probability that the ball so drawn is white.
Solution:
Give that bag I contains 8 white and 7 black balls and Bag II contain 5 white and 4 black balls.
One ball from I bag randomly put in II bag.
So the possibility is that ball taken out from bag I is white.
Then probability that white ball is choosen from bag I
= 8/15
Now total number of balls in bag II = 5 + 1 = 6
Probability that white ball is choosen from bag II
= 6/10
When both events occurs together
∴ Probability = 8/15 × 6/10 = 48/150
Another possibility is that ball is choosen from bag I is black.
Then probability that black ball is choosen from bag
= 7/15
Now number of black ball in bag II = 4 + 1 = 5
∴ Probability that black ball is choosen = 5/10
Probability that both events happen together
= 7/15 × 5/10 = 35/150
∵ Both events are mutually exclusive so only one event can happen.
∴ Requried probability = 48/150 + 35/120
= 83/150

Question 19.
In answering a question on a multiple choice test, a student either company the answer or guesses or he knows the answer. Let 1/6 be the probability that he copy the answer and 1/3 be the probability that he guesses Assuming that a student who copy the answer will be correct with probability 1/8. What is the probability that the student knows the answer given that he answered it correctly.
Solution:
Probability that student guesses the answer
P(A) = 1/3
Probability that student copies the answer
P(B) = 1/6
Probability that student knows the answer
P(C) = 1 – 1/3 – 1/6
= 6−2−1/  6 = 6−36
= 3/6 = 1/2
Let E is the event that answer is correct

Probability that student knows the answer and answered correctly

Question 20.
A letter known to have come either from Tatanagar and Calcutta. On the envelope just two consecutive letters TA are visible, what is the probability the letter has come from.
(i) Calcutta
(ii) Tatanagar
Solution:
Let E₁ be the event that letter came from Calcutta
and E₂ letter came from tatanagar
A = event that just two letters TA are visible.

Question 21.
A manufacturer has three machine operators A, B and C. The first operator A productes 1% defective items, whereas the other two operators B and C produce 5% and 7% defective items respectively. A is on the job for 50% of the time, B on the job for 30% of the time and C on the job for 20% fo the time. A defective item is produced. What is the probability that it was produced by A?
Solution:
Let E₁ = Product by machine A
E₂ = Product by machine B
E₃ = Product by machine C
Then E₁,E₂ and E₃ are mutually exclusive events

Question 22.
The random variable X has a probability distribution P(X) of the following from, where k is some number

(i) determine the value of k
(ii) find P(x < 2), P(x ≤ 2) and P(x ≥ 2)
Solution:
According to question
Probability distribution of X is

Question 23.
A random variable X can take all non-negative values and the probability that X take the value r is proportional to ar where (0 < α < 1). Find P(X = 0).
Solution:

Question 24.
Let X be random variable which assumes value x₁, x₂, x₃, x₄ such that
2P(X = x₁) = 3P(X = x₂) = 4P(X = x₃) = 5P(X = x₄)
find the probability distribution of X.
Solution:
Given 2P(X = x₁) = 3p(x = x₂) = P(X = x₃)
= 5 P(X = x₄) = K

Question 25.
A fair coin is tossed until a head or five tails occur. If X denotes the number of tosses of the coin, find the mean of X.
Solution:
Given X denotes the number of coins. Coins is tossed until a head or five tails accur therefere, it is clear if on X = 1, head comes then the process will be stopped and if tail comes then coin will be tossed second time. Clearly it will be repeated again and again till 5 tails come maximum.
Then the value of X will be 1,2, 3,4, 5
S = {H, TH, TTH, TTTH or TTTTH, TTTTT}
∴ Probability that head comes in first throw
P(X = 1) = 1/2
Similarly

SabDekho

The Complete Educational Website

Bihar Board

UP Board

IAS Notes

Jac Board

MP Board

सम्पूर्ण व्याकरण

जीवनी

निबंध

टेन्स

सामान्य ज्ञान

Rajasthan Board

NCERT Solutions in Hindi

NCERT Solutions in English